コスニタの定理
三角形におけるコスニタの定理(コスニタのていり)は、ある3本の線が共点であるという定理である。
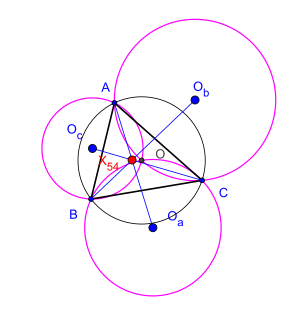
三角形 の外心を とし、三角形 , 三角形 , 三角形 の外心を とする。このとき「3本の直線 , , は1点で交わる」というのがコスニタの定理である[1]。この定理の名前はルーマニアの数学者 Cezar Coşniţă に由来する[2]。
上記の3本の線の交点はジョン・リグビーによってコスニタ点と命名されている。この点は九点円の中心の等角共役点になっている[3][4]。この点は Encyclopedia of Triangle Centers において として登録されている[5][6]。この定理はダオの六角形の周上の六円定理の特殊な場合である[7][8][9][10][11][12][13]。
参考文献
編集- ^ Weisstein, Eric W. "Kosnita Theorem". mathworld.wolfram.com (英語).
- ^ Ion Pătraşcu (2010), A generalization of Kosnita's theorem (in Romanian)
- ^ Darij Grinberg (2003), On the Kosnita Point and the Reflection Triangle. Forum Geometricorum, volume 3, pages 105–111. ISSN 1534-1178
- ^ John Rigby (1997), Brief notes on some forgotten geometrical theorems. Mathematics and Informatics Quarterly, volume 7, pages 156-158 (as cited by Kimberling).
- ^ Clark Kimberling (2014), Encyclopedia of Triangle Centers Archived 2012-04-19 at the Wayback Machine., section X(54) = Kosnita Point. Accessed on 2014-10-08
- ^ a b “ENCYCLOPEDIA OF TRIANGLE CENTERS X54”. faculty.evansville.edu. 2024年3月26日閲覧。
- ^ Nikolaos Dergiades (2014), Dao’s Theorem on Six Circumcenters associated with a Cyclic Hexagon. Forum Geometricorum, volume 14, pages=243–246. ISSN 1534-1178.
- ^ Telv Cohl (2014), A purely synthetic proof of Dao's theorem on six circumcenters associated with a cyclic hexagon. Forum Geometricorum, volume 14, pages 261–264. ISSN 1534-1178.
- ^ Ngo Quang Duong, International Journal of Computer Discovered Mathematics, Some problems around the Dao's theorem on six circumcenters associated with a cyclic hexagon configuration, volume 1, pages=25-39. ISSN 2367-7775
- ^ Clark Kimberling (2014), X(3649) = KS(INTOUCH TRIANGLE)
- ^ Nguyễn Minh Hà, Another Purely Synthetic Proof of Dao's Theorem on Sixcircumcenters. Journal of Advanced Research on Classical and Modern Geometries, ISSN 2284-5569, volume 6, pages 37–44. MR....
- ^ Nguyễn Tiến Dũng, A Simple proof of Dao's Theorem on Sixcircumcenters. Journal of Advanced Research on Classical and Modern Geometries, ISSN 2284-5569, volume 6, pages 58–61. MR....
- ^ The extension from a circle to a conic having center: The creative method of new theorems, International Journal of Computer Discovered Mathematics, pp.21-32.










